I have a huge problem with a lot of the science-based theories of how Pokéballs capture Pokémon. The matter-to-energy theories mean that there would be city-leveling nuclear explosions every time we captured even the smallest of Pokémon. The transporter theory means that there is a facility some place that holds all the monsters that we capture, which in the end means that there is a giant zoo someplace or a very inhumane warehouse storing all these creatures.
I think that it’s best to Occam’s razor this part of the discussion, because that’s not really what I want to talk about.
The simplest solution to how Pokémon are stored is that they are shrunk.
They become mini-versions of themselves small enough to fit into a ball the size of a fist, or in the case of Pokémon Go, they are stored in your phone. And as someone who thinks about the world scientifically and logically, this frustrates the hell out of me.
We have two major problems here. First, we have the Law of Conservation of Mass and the science behind decreasing the electron Bohr radius. Both of which would make all the walking you have to do to hatch your Pokéeggs impossible. Let’s science the shit out of this to find out why.
Density
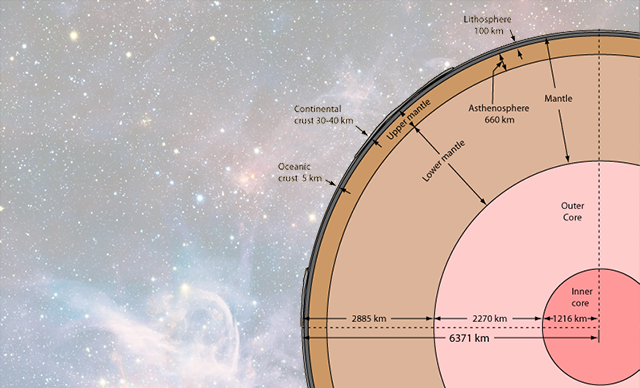
Everyone knows why boats float, right? We all know that large ocean liners weigh tons, but in the end they are less dense than the water that’s holding them up. There are two general forces acting on the boat: gravity and the buoyant force. The simplest way to explain this is that gravity pulls down and the buoyant force pushes up. And when the buoyant force and gravity are equal, the object in the water will float. This works in every fluid. And in its most basic form, the material that makes up Earth’s crust is a fluid.
There are other forces at work, that hold more dense objects to the surface of Earth, but if no other force acted on an object that was more dense than Earth’s crust, it would sink (albeit very slowly) to the mantle then to the core.
Given that, let’s look at some densities that we know. We know Earth’s crust has the average density of 2.2 g/cm³. The mantle sits at about 3.3 g/cm³. And the densest part of Earth is the core sitting at about 9.9 g/cm³. The densest known material on Earth is Osmium sitting at 22.6 g/cm³. And because it will be important later, I should mention that the world record for curling (lifting with your bicep) is 155 kg, made by this guy on YouTube. (It’s a weird video, worth watching for the oddity if nothing else.)
 Law of Conservation of Mass
Law of Conservation of Mass
Did you know that nothing actually gets lighter? If we are on a diet and losing weight, the mass doesn’t just disappear. We excrete it out in some fashion when we sweat or pee.
That same principle applies to everything in the universe. Nothing ever just disappears. It is actually reformed into something else.
When you light hydrogen on fire, mixing it with oxygen, we know that it becomes water. If you expand a balloon with air, you’re not making the balloon heavier. What you’re doing is changing it. The first example is a chemical change and the second is a density change. We will be focusing on the second part.
In my area, the most common Pokémon has to be Rattata. According to the Bulbapedia, a Rattata weighs in at a nominal 3.5 kg and stands at 30 cm. I’m going to assume that it’s about half that width and about twice that in length, giving it the volume of 27,000 cm³ and the density of about 0.129 g/cm³.
If we were to shrink that Rattata to the size of a Pokéball, the mass will not change, but the volume will. The volume of a Pokéball is roughly the same as an orange. An orange is on average is 10 cm in diameter (5 cm radius), giving it the volume of 523.59 cm³. Squeezing a Rattata into a space of 523.59 cm³ will give it the density of 6.68 g/cm³.

See where I’m going with this?
A caught Rattata would be denser than the mantle of Earth, and a Jigglypuff would be roughly just as dense as the inner core. A Wigglytuff or Sandshrew will be more dense than Osmium if jammed into a Pokéball.
And remember our world-record holder from earlier? Well, he will have trouble lifting a Golduck, and don’t even ask him to lift up a Pokéball carrying a Slowbro. Even if every Pokémon you ever collected was Ghastly, it’s going to add 25 kg to your phone before you reach your maximum inventory. For those who weigh things in pounds, that’s adding an instant 55 lbs to your jeans.
Literally, as I was writing that last paragraph, a friend of mine messaged me a picture of his recently captured Ponyta, proclaiming, “I found my favorite Pokémon!” To which I replied, “And your pants just became 82 lbs heavier. Welcome to science, motherf*cker!”
Breaking the Bohr radius
Despite all the issues we would have just lifting our trousers and giving a new definition to saggy pants, the only way to shrink a Pokémon to the size of a Pokéball and retain all of its current properties is to somehow reduce the distance between its electrons — its Bohr radius, if you will. Right now the only people on Earth sciencing the shit out of reducing the Bohr radius are those operating the Large Hadron Collider.
Yeah, that means that quantum physicists haven’t even figured out that out, Professor Oak. Good try.
What do you think? Science isn’t science unless it’s proven wrong. How would you science the shit out of this? Let me know in the comments below.






Published: Jul 14, 2016 08:55 am